Networks of queueing-inventory systems

Working Groups: Lehrstuhl Angewandte Analysis

Collaborators (MAT): Dr. Sonja Otten

Collaborators (External): Prof. Dr. Hans Daduna, Dr. Ruslan Krenzler

Description

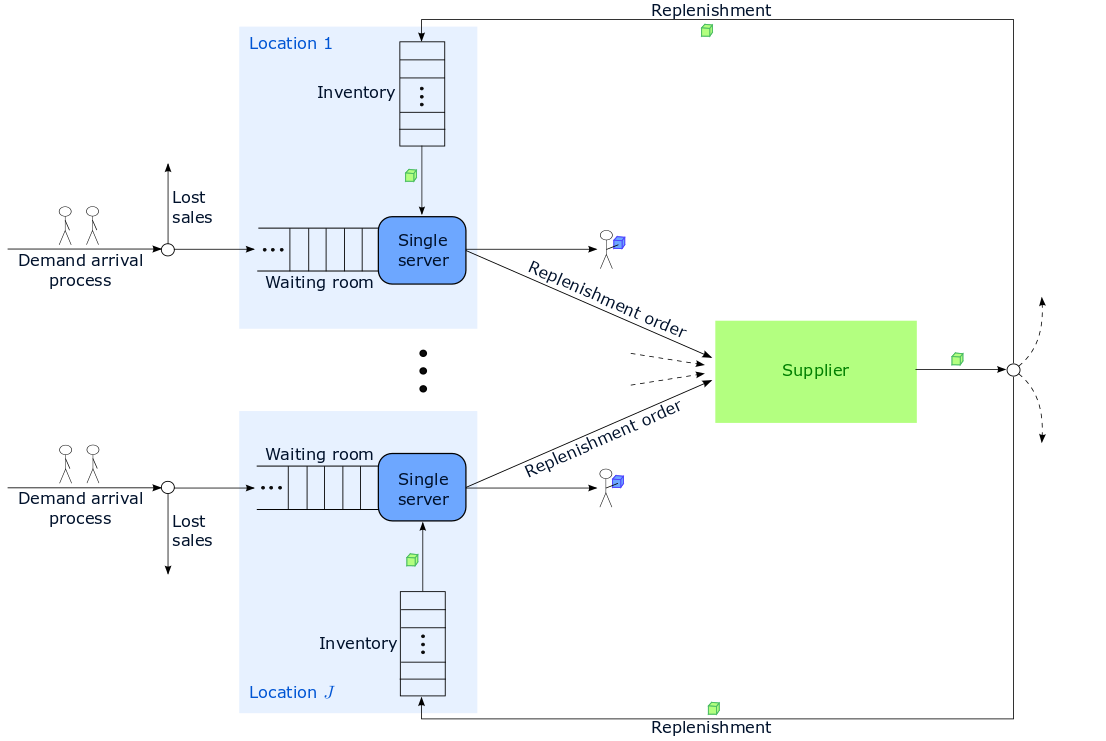
Production processes are usually investigated using models and methods from queueing theory. Control of warehouses and their optimization rely on models and methods from inventory theory. Both theories are fields of Operations Research, but they comprise quite different methodologies and techniques. In classical Operations Research queueing and inventory theory are considered as disjoint research areas. On the other side, the emergence of complex supply chains (≡ production-inventory networks) calls for integrated production-inventory models as well as adapted techniques and evaluation tools. We consider several networks of production-inventory systems as shown in the Figure, which consists of parallel production systems (single servers) at different locations each with an attached local inventory, and a supplier, which produces raw material (discrete units) to replenish the local inventories.

Integrated approaches to model production-inventory systems have been developed over the last decade and it turned out that the problem of determining e.g. steady states of the systems usually results in either large simulation experiments or in using heuristic decomposition-aggregation methods or in solving the global balance equations numerically. Our methodological approach constitutes an alternative to simulations and/or heuristic decomposition-aggregation techniques. We develop Markovian stochastic models of several production-inventory systems, which are smooth enough to be amenable to solving the steady state problem explicitly with closed form expressions for the stationary distribution. Moreover, for most of the integrated production-inventory systems the obtained steady state is of so-called product form, which reveals a certain decoupling of the components of the system for long time behaviour. This product form structure of the joint stationary distribution is often characterised as the global process being separable. It is an important (but rather rare) property of complex systems. The simple structure of these steady states allows to apply product form calculus, a widely used tool, which provides access to easy performance evaluation procedures.

References

[1] S. Otten, R. Krenzler, H. Daduna. Models for integrated production-inventory systems: steady state and cost analysis. International Journal of Production Research, 54(20):6174-6191, 2016. doi: 10.1080/00207543.2015.1082669.
[2] S. Otten. Integrated Models for Performance Analysis and Optimization of Queueing-Inventory-Systems in Logistic Networks. Phd thesis, Universität Hamburg, 2018. Available at ediss.sub.hamburg.
[3] S. Otten, R. Krenzer, H. Daduna. Separable models for interconnected production-inventory systems. Stochastic Models, 36(1):48-93, 2020. doi: 10.1080/15326349.2019.1692667.
[4] S. Otten. Load balancing in a network of queueing-inventory systems. Ann Oper Res, 2022. doi: 10.1007/s10479-022-05017-3.