Finite Sections of Aperiodic Schrödinger Operators

Working Groups: Lehrstuhl Angewandte Analysis

Collaborators (MAT): Fabian Gabel, M. Sc., Prof. Dr. Marko Lindner, Riko Ukena, M. Sc.

Collaborators (External): Dennis Gallaun, Julian Großmann

Description

Discrete Schrödinger operators are used to describe physical systems on lattices and, therefore, play an important role in theoretical solid-state physics. For a fixed \(p \in [1,\infty]\), consider the Schrödinger operator \(H \colon \ell^p(\mathbb{Z}) \to \ell^p(\mathbb{Z})\) given by

\[ (H x)_n = x_{n + 1} + x_{n - 1} + v(n) x_nn \in \mathbb{Z}, \](1)

and its one-sided counterpart \(H_+ \colon \ell^p(\mathbb{N}) \to \ell^p(\mathbb{N})\) given by

\[ (H_+ x)_n = x_{n + 1} + x_{n - 1} + v(n) x_n\;,n \in \mathbb{N}, \quad x_0 = 0\;. \] (2)

Based on Definitions \((1)\) and \((2)\), one can associate \(H\) and \(H_+\) with infinite tridiagonal matrices \(A = (a_{ij})_{i,j \in \mathbb{Z}}\) and \(A_+ = (a_{i,j})_{i,j \in \mathbb{N}}\).

Looking at the corresponding infinite linear system of equations

\[ A x = b \quad\text{and}\quad A_+ y = c \]

it is interesting to know if the solutions \(x\) and \(y\) to theses systems can be computed approximately by solving the large but finite linear systems

\[ A_m x^{(m)} = b^{(m)} \quad\text{and}\quad (A_+)_m y^{(m)} = c^{(m)} \]

and letting \(m \to \infty\). This is the main idea of the Finite Section Method (FSM). In order to assure the applicability of the above procedure, one investigates further properties of the operator \(A\), the sequence \((A_n)\) and its one-sided counterparts. In particular, Fredholm Theory, spectral theory and the concept of limit operators play a central role in this investigation [6].

This research project deals with the investigation of the applicability of the FSM to problems surging from discrete Schrödinger Operators and its interplay with spectral theory, see our works [1-5]. A famous example for theses operators is the so called Fibonacci-Hamiltonian, see [3], where the potential \(v\) is given as

\[ v(n) := \chi_{[1 - \alpha, 1)}(n \alpha \operatorname{mod} 1)\;, \quad n \in \mathbb{Z}. \]

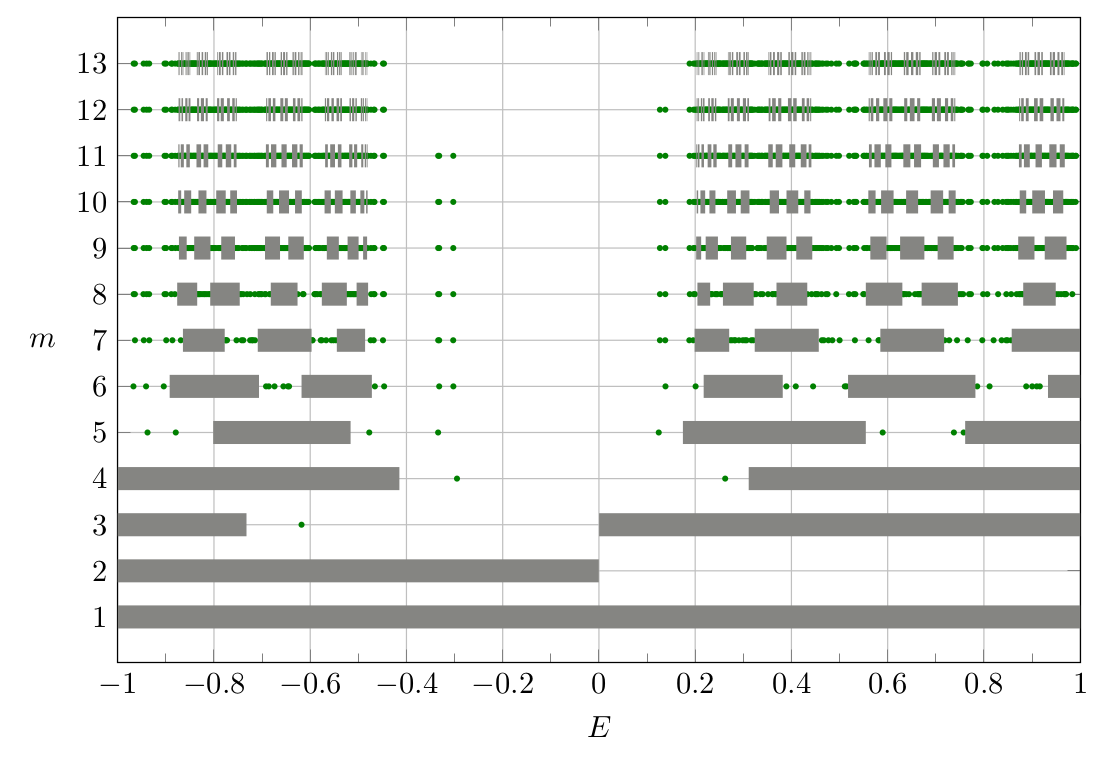
For this particular example, the central objects of investigation are periodic approximations \((A_m)\). It is crucial to assure that the spectrum of these approximations eventually avoids the point \(0\) for larger numbers of \(m\). The following graph shows approximations of the spectra of the one-sided Fibonacci Hamiltonian on \(\ell^2(\mathbb{N})\).

References

[1] F. Gabel, D. Gallaun, J. Großmann, M. Lindner, and R. Ukena. Analysis Code for Finite Sections of Periodic Schrödinger Operators. Publisher: TUHH Universitätsbibliothek. 2021. doi:10.15480/336.3828.

[2] F. Gabel, D. Gallaun, J. Großmann, M. Lindner, and R. Ukena. Finite Sections of Periodic Schrödinger Operators. To appear in: Operator Theory and Applications. 2021. arXiv:2110.09339v2.

[3] F. Gabel, D. Gallaun, J. Großmann, and R. Ukena. Finite section method for aperiodic Schrödinger operators. 2021. arXiv:2104.00711v1.

[4] F. Gabel, D. Gallaun, J. Großmann, M. Lindner, and R. Ukena. Spectral Analysis Code for Aperiodic Schrödinger Operators. Zenodo. 2021. doi:10.5281/zenodo.5201653.

[5] F. Gabel, D. Gallaun, J. Großmann, and R. Ukena. Spectral approximation of generalized Schrödinger operators via approximation of subwords. 2022. 2209.11613v1.

[6] M. Lindner. Infinite Matrices and their Finite Sections. Frontiers in Mathematics. Birkhäuser Basel, 2006. doi:10.1007/978-3-7643-7767-0.